Homework 6
CM3110
-
(adapted from Geankoplis 2nd edition 4.1-1, page 317 Calculate the heat
loss per square meter of surface area for an insulating wall of a food
cold-storage room where the outside temperature is 299.9K and the
inside temperature is 276.5K. The wall is composed of 25.4 mm of
corkboard having a thermal conductivity of 0.0433 W/(m K). SOLUTION
-
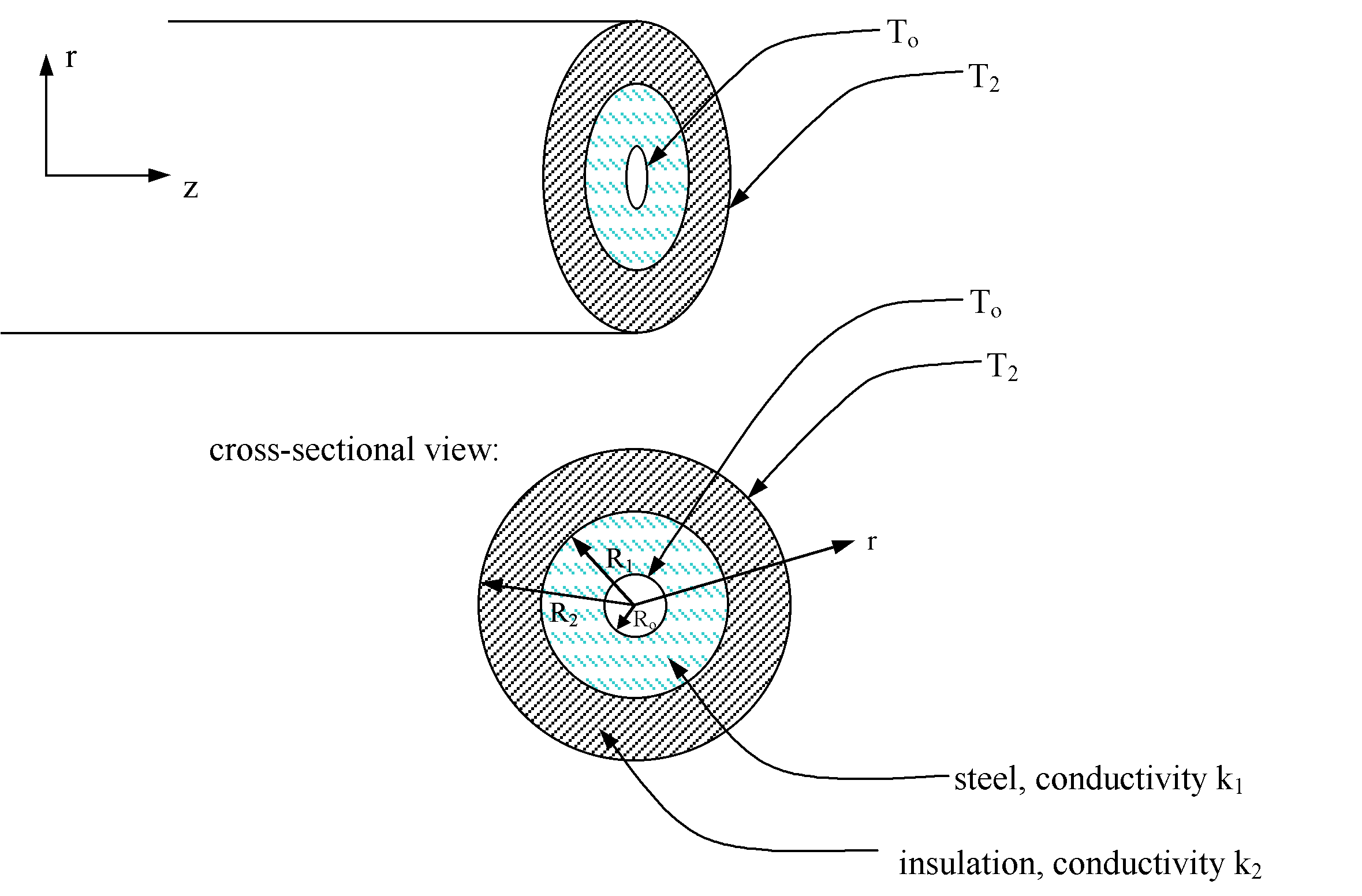
The inside wall of an insulated pipe is held at To and the
surface
on the outside wall of the insulation is held at T2, where To
>
T2 (see below). Assuming that the pipe is very long and that
there is no heat loss out the ends of the pipe, calculate the steady
state
temperature profile in the radial direction. Hint: within each
material,
solve for T(r) the same way as we did in class. To solve for the
unknown
temperature, match the solutions at the boundaries, i.e. heat flux is
the
same in both materials at r = R1. SOLUTION
- In radial heat flux in a pipe, if the thermal conductivity
varies linearly with temperature, show that the result for heat flux is
the same as the result obtained for constant thermal conductivity if
the
value of conductivity used is the average of the values obtained at the
boundaries, km = 0.5(k(T1)+k(T2).). SOLUTION
- (adapted from Geankoplis 2nd edition 4.2-2) A cooling coil of 1.0
ft of 304 stainless steel tubing having an inside diameter of 0.25 in
and and outside diameter of 0.40 in is being used to remove heat from a
bath. The temperature at the inside surface of the tube is 40oF
and it is 80oF on the outside. The thermal
conductivity of 304 stainless steel is a linear function of temperature
with a slope of 7.78 X 10-3 and an intercept of 7.75 for k
given in units of btu/(h ft oF) and temperature given in
units of oF. Calculate the heat removal in btu/s and
in watts. SOLUTION
- (adapted from Geankoplis 2nd edition 4.3-2) A wall of a
furnace 0.244 m thick is constructed of material having a thermal
conductivity of 1.30 W/(m K). The wall will be insulated on the
outside with material having an average thermal conductivity of 0.356
W/(m K). The thickness of the insulation must be such that the
heat loss from the furnace will be equal to or less than 1830 W/m2.
The inner surface temperature is 1588 K and the outer surface
temperature is 299K. Calculate the thickness of insulation
required. SOLUTION
- (adapted from Geankoplis 2nd ed 4.3-6) A glass window with
an area of 0.557 m2 is installed in the wooden outside wall
of a room. The wall dimensions are 2.44 X 3.05 m. The wood
has a thermal conductivity of 0.1505 W/(m K) and is 25.4 mm
thick. The glass is 3.18 mm thick and has a thermal conductivity
of 0.692 in the same units. The inside room temperature is 299.9
K (26.7oC) and the outside air temperature is 266.5 K.
The convection heat-transfer coefficient hi on the inside
wall of the glass and of the wood is estimated to be 8.5 W/(m2
K) and the outside heat-transfer coefficient ho is also
estimated to be 8.5 in the same units for both surfaces.
Calculate the heat loss through the wooden wall, through the glass, and
the total heat loss. SOLUTION

