Homework 9
CM3110
Heat Exchanger Effectiveness
Shell-and-tube heat exchangers
- You are asked to design a double-pipe heat exchanger for
an application that will continuously heat a process stream (Cp=4.85kJ/kg
K, mass flow rate=0.584 kg/s) from 25oC to 95oC.
The heat-transfer fluid will be Thermatic® (Cp=12.8
kJ/kg
K), which will be fed at 125oC. The mass flow rate of the
Thermatic®
is not specified and must be chosen in the design process. The
viscosities
of the process fluid and of the Thermatic® may be assumed to be
constant
(not a function of temperature). Describe how you will
estimate the overall heat-transfer coefficient
U for the heat exchanger. Please write down all equations
you
will use and indicate how you will use them. Please list separately all
the physical property data you will need and how you will determine
any quantities (diameters, velocities, etc.) in your equations. If
your solution is iterative, please outline your iterative
strategy. (SOLUTION)
- Design a double-pipe heat exchanger for
an application that will continuously heat a process stream (Cp=4.85kJ/kg
K, mass flow rate=0.584 kg/s) from 25oC to 95oC.
The heat-transfer fluid will be Thermatic® (Cp=12.8
kJ/kg
K), which will be fed at 125oC. The mass flow rate of the
Thermatic®
is not specified and must be chosen in the design process. The
viscosities
of the process fluid and of the Thermatic® may be assumed to be
constant
(not a function of temperature). (no solution provided)
- (Based on example 4.9-2 from Geankoplis 3rd edition page 275)
Water flowing at a rate of 0.667 kg/s enters a countercurrent,
double-pipe heat exchanger at 308K and is heated by an oil stream
entering at 383K at a rate of 2.85 kg/s (cp=1.89 kJ/(kg
K). The overall heat transfer coefficient of the heat exchanger
is 300. W/(m2 K) and the heat transfer area in the exchanger
is 15.0 m2. Calculate the heat-tranfer rate and the
exit water temperature, first using heat exchanger effectiveness (no
solution given; plot for effectiveness versus NTU=UA/Cp,min
is given in McCabe et al. p445 or in Geankoplis 3rd ed p274) and then
without using the effectiveness.
How well do your answers compare? Note Geankoplis 3rd edition has
the solution to the problem when effectivenes is used. (SOLUTION)
- From Figure 4.9-7 on page 274 of Geankoplis 3rd edition (given in
lecture notes), at what values of
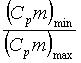 and NTU is it better to use co-current flow instead of counter-current
flow? Please explain your answer. (SOLUTION)
and NTU is it better to use co-current flow instead of counter-current
flow? Please explain your answer. (SOLUTION)
- (based on Geankoplis 3rd edition 4.8-4) Steam at 1.00 atm
pressure (absolute) and 100.oC is condensing on a bank of 5
vertical tubes each 0.305 m high and having an outer diameter of 1.00
in. The tubes are arranged in a bundle spaced far enough apart so
that they do not interfere with each other. The surface
temperature of the tubes is 97.78oC. Calculate the
average heat-transfer coefficient and the total mass flow rate of
condenstate (kg/h). (SOLUTION)
- (based on Geankoplis 3rd edition 4.9-1) A 1-2 shell-and-tube heat
exchanger with one shell pass and two tube passes is used to heat a
cold fluid from 37.8oC to 121.1oC by using a hot
fluid entering at 315.6oC. The temperature of the hot
fluid leaving the exchanger is measured to be 148.9oC.
Calculate the log-mean temperature difference in the exchanger and the
mean temperature difference in the exchanger. Comment on these
answers - why are they different? Are they different in the way
you expected? (SOLUTION)
- (based on Geankoplis 3rd edition 4.9-2) Oil flowing at a rate of
5.04 kg/s (mean Cp=2.09 kJ/(kg K)) is cooled in a 1-2
shell-and-tube heat exchanger from 366.5K to 344.3K by water flowing at
2.02 kg/s entering at 283.2K. The overall heat-transfer
coefficient based on the outer area is 340. W/(m2 K).
Calculate the area required. (SOLUTION)
- (based on Geankoplis 3rd edition 4.9-4) Hot oil at a flow rate of
3.00 kg/s (heat capacity Cp=1.92 kJ/(kg K)) enters an
existing counterflow exchanger at 400.K and is cooled by water entering
at 325 K (under pressure) and flowing at a rate of 0.70 kg/s. The
overall heat-transfer coefficient is 350. W/(m2 K) and the
heat-transfer area is 12.9 m2. Calculate the
heat-transfer rate and the exit oil temperature. (SOLUTION)
- A countercurrent, double-pipe heat exchanger (inner heat transfer
area
= 42.6 m2, overall heat-transfer coefficient based on the
area
given = 340. W/(m2 K)) was designed to cool a vegetable oil
(mean
heat capacity = 5.62 kJ/(kg K), flow rate = 5.3 kg/s) from 120.oC
to 85oC using city water as the cooling fluid. The city
water
is supplied at a temperature of 18oC. (SOLUTION)
- What is the outlet temperature of the city water for the
conditions
given?
- After some years of use we know that the heat exchanger will
become
fouled.
If we assume that the water temperatures (both inlet and outlet) and
all
flow rates do not change, what will be the new overall heat transfer
coefficient
and outlet temperature of the oil after fouling? The ratio of inner to
outer diameter for the inside pipe of the heat exchanger is 0.887.
- How could we adjust the operating conditions of the heat
exchanger to
prevent
this degradation of performance of the unit?
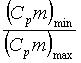 and NTU is it better to use co-current flow instead of counter-current
flow? Please explain your answer. (SOLUTION)
and NTU is it better to use co-current flow instead of counter-current
flow? Please explain your answer. (SOLUTION)