|
|
|
|
|
|
| F3.2 | F3.6 | F4.0 | F4.5 | F5.0 |
|
|
|
|
|
|
| F5.6 | F6.4 | F7.1 | F8.0 | F9.0 |
| Click on the image to see a larger one | ||||
When a lens focuses on a subject at a distance, all subjects at that distance are sharply focused. Subjects that are not at the same distance are out of focus and theoretically are not sharp. However, since human eyes cannot distinguish very small degree of unsharpness, some subjects that are in front of and behind the sharply focused subjects can still appear sharp. The zone of acceptable sharpness is referred to as the depth of field. Thus, increasing the depth of field increases the sharpness of an image. We can use smaller apertures for increasing the depth of field.
The following shows an example. The lens focuses at the middle between the 3 inch and 4 inch marks. Thus, the 3 inch and 4 inch marks are sharp in all images. The 5 inch mark is not very sharp at F3.2, and is improved as the lens closes down to F3.6. Then, it becomes sharp in all subsequent images. The 6 inch and 7 inch marks are not sharp until F5.0 and F6.4, respectively. The 8 inch mark becomes reasonably sharp when the lens closes down to F8.0. The 9 inch and 10 inch marks are not sharp in all images; but, they become sharper as the lens closes down. For the foreground, the 2 inch mark is acceptable at F3.2 and becomes "focused" at F4.0. The 1 inch mark is not sharp until F5.6, and the lead of the ruler becomes reasonably sharp at F7.1. As you can see, the range of sharpness (i.e., depth of field) gets larger as the aperture gets smaller. Therefore, use a smaller aperture if a greater depth of field is needed. Please check the Aperture-Priority Mode to know more about the use of aperture and its impact on depth of field.
|
|
|
|
|
|
| F3.2 | F3.6 | F4.0 | F4.5 | F5.0 |
|
|
|
|
|
|
| F5.6 | F6.4 | F7.1 | F8.0 | F9.0 |
| Click on the image to see a larger one | ||||
Suppose the lens focuses on the yellow dot as shown in the figure below. This subject generates a yellow dot on the image plane. Once focused, all subjects that have the same subject-lens distance as that of the yellow dot will appear sharp. Now, consider a white dot that is behind the yellow dot (i.e., with larger subject-lens distance). Since it is out of focus, it will not produce a sharp white dot image. Instead, its image is formed somewhere in front of the image plane. On the image plane, the image of this white dot is a circle as shown below. This circle is usually referred to as a circle of confusion. As the subject-lens distance increases, the size of this circle increases. The same holds true for a subject in front of the yellow dot (e.g., the green dot in the following figure). Since these circles of confusion are actually out of focus images of subjects, if we can reduce the size of circle of confusion, we can increase the sharpness of the resulting image. But, how?
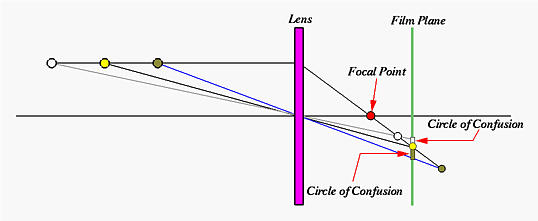
It turns out to be very simple. Since circles of confusion are formed by light rays passing through the lens tube, the size of a circle of confusion is proportional to the amount of light that can pass through the lens tube. This means smaller (resp., larger) circles of confusion will be formed if less (resp., more) light can pass through. Restricting how much light can pass through the lens is the function of the diaphragm in the lens tube that sets the aperture values. Therefore, a smaller aperture means a smaller diaphragm opening, which, in turn, means allowing less light to strike the film/CCD plane. Thus, we have smaller circles of confusion and, as a result, a sharper image!
In addition to closing down the diaphragm (i.e., smaller aperture), we can also focus at the hyperfocal distance. If a lens focuses at infinity, the depth of field starts at somewhere in front of the lens and extends to infinity. More precisely, from that point on, the scene appears sharp, and subjects between that point and the lens are out of focus. The distance from the lens to that point is referred to as the hyperfocal distance. In the figure below, the hyperfocal distance is marked with a thick red line.

After determining the hyperfocal distance, we can point the camera at this distance. The resulting depth of field starts from halfway of the hyperfocal distance and extends to infinity. This is, in fact, the maximum depth of field we can get. The concept is shown in the figure below.
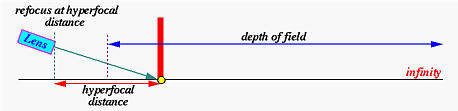
Why is this hyperfocal distance important? When shooting a distant subject or scene, many people point the camera to that subject(s). The result may be satisfactory; however, in many cases the foreground looks out of focus. This is, of course, because of insufficient depth of field. Should this happen, the above technique becomes very useful: (1) focus at infinity; (2) determine the hyperfocal distance; (3) refocus at the hyperfocal distance; and (4) take a photo.
Please note that hyperfocal distance is a function of the aperture being used. More precisely, changing aperture changes hyperfocal distance.
Unfortunately, the answer is "no." As the light rays passing the lens tube and the diaphragm, some may be diffracted as shown in the figures below. If the diaphragm is large (i.e., a large aperture), because the proportion of the diffracted light and the non-diffracted light is so small and is negligible, diffraction does not contribute to the loss-of-quality very much. See the left figure below. However, when the diaphragm is small (i.e., a small aperture), the amount of light that can pass through the diaphragm is reduced and hence the proportion of the diffracted and non-diffracted light becomes significant. As a result, the quality of the image is also reduced. See the right figure below. Therefore, closing the diaphragm (i.e., using small apertures) all the way down to the smallest aperture may not increase the quality of an image. In general, the quality of a lens increases as diaphragm closes down. This improvement will reach certain point. After this, quality goes down because of the impact of diffraction.
