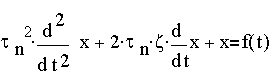
Standard Form:

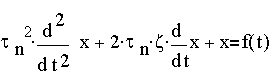
Further, consider the case where

( 0 ) = 0

Then the characteristic equation is :

   whose roots are given by:

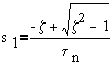
      and

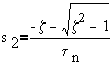
Case 1:     Both roots are real and negative ( and thus stable)

Using initial condtions,

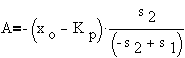
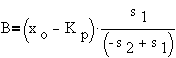
or

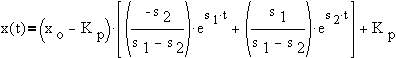
Case 2:   Repeated real roots

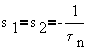
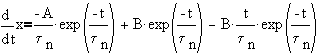
Using initial conditions,

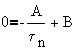
or

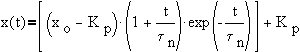
Case 3:  Roots are complex conjugates

         and 

           and 

Thus,

 
Using initial conditions,

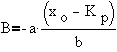
Alternatively, we can use the sin of sums formula,

comparing right hand side with,

then

          and 

from which,

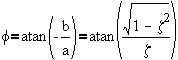
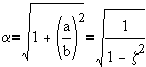
so, an alternative form for x(t) is given by

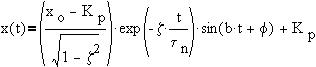
where,         is the frequency
and         is the phase shift

Case 4: z=0   Pure imaginary roots

Case 5: z<0  Unstable roots

Derivation of Overshoot, Decay Ratio and Frequency (Underdamped Systems) :
 

a) Overshoot:

First, calculate the time when the peaks occur. To do so, obtain dx/dt, and then find the value of t which makes it zero.

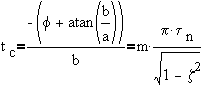
for upper peaks, 

The overshoot occurs at the first upper peak, i.e.

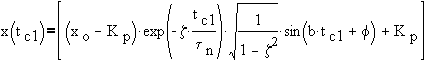
but,

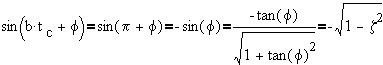
so the overshoot is given by
 
 

b) Decay ratio

Compare the value at the first peak and the second upper peak. The second upper peak occurs at t_c3

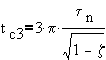
thus, the decay ratio is given by,

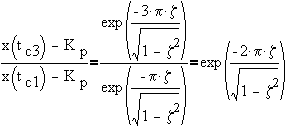
c) Frequency

The period of oscillation is the amount of time between two upper peaks

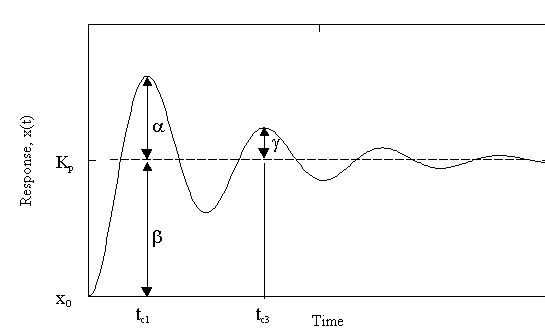
Overshoot =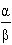 
Decay Ratio= 
Frequency= ( in rads/sec )

This page is maintained by Tomas B. Co (tbco@mtu.edu). Last revised 12/2//99.

     Tomas B. Co
     Associate Professor
     Department of Chemical Engineering
     Michigan Technological University
     1400 Townsend Avenue
     Houghton, MI 49931-1295

Back to Homepage